How Do I Calculate Mortgage Repayments?

It would be easy to figure out a mortgage payment if the numbers didn’t change over time. Unfortunately for us, they do—quite a bit. Banks need to make money off the money they lend, so they charge interest on a loan. Mortgage interest is basically the fee the bank charges you to borrow money.

There’s an old story that Albert Einstein called compound interest the “most powerful force in the universe.” While we’re not sure if it’s worthy of that much praise, it is quite powerful. The word “compound” makes things more difficult for us. If you borrow £10,000 for 10 years at 2% simple interest, you’ll pay £200 in interest each year: that's quite simple. However, if you borrow with compound interest, we have to calculate the interest every time you make a payment.

Mortgages in the UK use compound interest, so the math goes like this:

1. You borrow £10,000 at 2% interest for five years, with yearly payments of £2,121.58 (You can use a calculator to check this. We use Excel's built-in PMT function).
2. The first year, you owe the bank £10,000. You’ll make a payment of £2,121.58. You’re paying 2% interest, so £200 of that payment is interest, the other £1,951.58 is principal. (The principal is the amount you borrowed originally) Why is the difference between interest and principal important? Interest goes straight to the bank, but you subtract the principal from the amount you owe next year: £10,000-£1,951.58=£8,078.42.
3. The second year, you owe the bank less (£8,078.42). You’ll still make a payment of £2,121.58, but you’ll pay less interest this time. 2% of £8,078.42 is £161.57, and the rest (£1,960.02) goes to the principal. Now you owe the bank £6,118.40.
4. Year three, you make the same payment of £2,212.58. This time, you pay interest of 2% on £6,118.40: this comes to £122.37. You now owe £4,119.18
5. Year four, repeat: 2% of £4,119.18 is £82.38. Now you owe £2,079.98.
6. Year five (finally!), you make the final payment: £2,079.98 plus 2% interest sums up to a neat £2,121.58. Notice how this is the exact size of your payment—that’s what makes the formula useful.

Tricky, right? This is also the reason interest rates are so important: if you had a 5% interest rate in the above example, you’d pay almost £1,000 more in interest. Imagine what would happen if it were a £400,000 mortgage over 25 years! (Hint: it’s not pretty)

We’ve been talking about fixed rates so far, where the interest rate doesn’t change. In a variable rate mortgage, your interest rate can change, often at the whim of the bank. Usually, this variable rate is determined by the Bank of England’s bank rate, plus two or three percent. On a standard variable rate, the lender has total control over your interest rate.

If you thought compound interest was tricky, variable rates are positively devilish. Most banks just quote a “cost for comparison:” this is an educated guess of what your average interest rate will be if you stay on that mortgage. These educated guesses are about as good as we can do: if you do figure out how to predict interest rates accurately, call us. (It’s very difficult.)

This is important because most mortgages have a fixed rate for a short period: 2-5 years, typically. The day your mortgage leaves this introductory rate, you’ll be paying a variable rate, and your payments can change every month!

For the maths-inclined among us, the mortgage payment formula isn’t that complicated. Just remember, this doesn’t account for variable rates, which can change.

You’ll need these numbers to get started:

• r = Annual interest rate (APRC)/12 (months)
• P = Principal (starting balance) of the loan
• n = Number of payments in total: if you make one mortgage payment every month for 25 years, that’s 25*12 = 300

Here’s the formula:

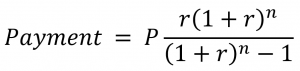
If we wanted to figure out the payment for an average mortgage, it might look like this:

• r = 0.033/12 = 0.00275 (This is 3.3% interest: you need to divide by 100 to make it a usable number for this formula.)
• P = £350,000
• N = 25*12 = 300 (One payment a month for 25 years)

If you can’t tell from the points above, this is a £350,000 mortgage at 3.3% APRC and a 25-year term.

Let’s plug those numbers into the formula:

And we'll simplify:

And that’s it! It may not be as easy as a calculator, but it's quite possible to do yourself.

If you enjoyed this article, don’t forget to subscribe for updates here. Thanks for reading!